Dean Flow Dynamics in Low-Aspect Ratio Spiral Microchannels (N. Nivedita, P. M. Ligrani, and I. Papautsky), Nature – Scientific Reports, Vol. 7, Article Number 44072, pages 1-10, March 2017.

Abstract

A wide range of microfluidic cell-sorting devices has emerged in recent years, based on both passive and active methods of separation.  Curvilinear channel geometries are often used in these systems, due to presence of secondary flows, which can provide high throughput and sorting efficiency.  Most of these devices have been designed on the assumption that there are two counter rotating Dean vortices, present in the curved rectangular channels, which exist in the state of steady rotation and amplitude.  In this work, we investigate these secondary flows in low aspect ratio spiral rectangular microchannels and define their development with respect to the channel aspect ratio and Dean number. This work is the first to experimentally and numerically investigate Dean flows in microchannels for Re > 100, and show presence of secondary Dean vortices beyond a critical Dean number. We further demonstrate the impact of these multiple vortices (>2) on particle and cell focusing. Ultimately, this work offers new insights into secondary flow instabilities for low-aspect ratio, spiral microchannels, with improved flow models for design of more precise and efficient microfluidic devices for applications such as cell sorting and micromixing.

Introduction

Inertial microfluidics has gained considerable interest in recent years for cell analysis^1,2 and sample preparation^2,5,6 on chip. Inertial microfluidic devices offer high throughput and efficiency, leading to reductions in cost and labor as compared with the conventional cell-sorting techniques such as fluorescence activated cell sorting (FACS),³ magnetic activated cell sorting (MACS),⁴  and filtration.^1,2  The key to this sorting approach is manipulation of geometry-induced hydrodynamic forces, which in turn provides continuous size-based sorting of cells in a flow-through manner.^6,7 The approach has been implemented using both straight and curved microchannels.^6,8-10

Inertial focusing of particles and cells in straight microchannels has been investigated extensively in recent years. It has been demonstrated that focusing is dependent on the balance of the shear-induced lift force (arising from the parabolic velocity profile) and the wall-induced lift force (arising from interactions with channel walls).^5-9 Under certain conditions in rectangular microchannels, a rotation-induced lift force, imposed on the suspended particles/cells, further dominates migration and drives microparticles toward the centers of the larger walls. We recently introduced the two-stage model of particle-focusing⁵ in straight microchannels that describes the interplay of these forces.^5-9 This concept of particle focusing gets complicated even further when a curvature is added to the geometry. A curvature results in development of secondary flow which induces Dean force (F_D) that can be used to further manipulate the focusing positions of suspended cells and microparticles.^10-14

A large number of cell-analysis devices^7,9-15 is based on the concept of secondary flows in curved microchannels. Majority of these devices are of spiral geometry and are designed on the assumption that the secondary flow in spiral microchannels leads to two counter-rotating vortices which are similar in amplitude and lead to the net Dean force experienced by the particles or cells.^10-14 These spiral cell-sorting devices rely on the balance of the net hydrodynamic forces (F_L) and Dean force to separate cells according to their size.^10-14,16 The result of this force balance is a single equilibrium position near the inner channel wall (Fig. 1a). Spiral devices based on this concept have been used by us^10-12 and others^13,14 for multitude cell sorting applications, including separation of neuroblastoma cells and C6 glioma cells¹⁰, sorting WBCs and RBCs¹² and separating polymorphonuclear leukocytes and mononuclear leukocytes¹⁴.

The assumption of two counter-rotating vortices is partially supported by various fluid mixing studies, which although quite detailed, are primarily numerical in nature and are often confined to low Reynolds numbers, Re < 20.^15-20 For example, the study of fluid mixing in curved microchannels by Howell et al.¹⁵ and convective vortex based mixing in spiral microchannels by Sudarshan et al.⁴¹, focus on lower Re (Re<18). However, at higher Re this assumption of two-counter rotating vortices falters since the focusing positions of cells vary from the predicted ones. In fact, we observed this variation while infusing a diluted blood sample in spiral microchannels for blood cell sorting (discussed in later sections). At higher Re (Re>100), RBCs focused closer to the outer channel wall/concave wall instead of focusing closer to the inner channel wall /convex wall (as predicted by the assumption) in a spiral sorting device. We attributed this behavior to the anomaly in the assumption of two counter rotating vortices and lack of physical understanding of the fluid flow dynamics in spiral microchannels at higher Re.

In this work, we provide a systematic experimental investigation of the fluid flow dynamics in low aspect ratio rectangular spiral microchannels which are widely used as highly efficient cell-sorters or micromixers. For the first time we demonstrate the presence of multiple pairs of secondary flow vortices (secondary Dean vortices) in these microchannels at high Re. We also introduce a non-dimensional parameter, critical Dean number (De_C), to represent a threshold for the onset of these secondary Dean vortices. This work offers insight into the phenomenon of development of secondary flows in spiral microchannels and improves the understanding of the concept of particle focusing in spiral devices. Additionally, this work also paves way into understanding the reason behind the need for a certain range of Dean number for cell focusing in current sorting techniques. Furthermore, the concept presented can potentially assist in manipulation of the interaction of multiple vortices and other inherent fluid forces to achieve higher efficiency and selectivity in cell sorting.

Results

Evolution of Dean vortices

The first conclusive research to analyze flow in curved macrochannels was done by Dean^21,22 in 1927. He showed that in curved pipes (circular cross-section), the laminar Poiseuille flow is subjected to centrifugal force (F_CF) (Fig. 1a).  This external force disturbs the parabolic profile of the laminar flow/primary flow and the maximum point of velocity distribution shifts from the center of the channel towards the concave wall of the channel. This shift causes a sharp velocity gradient to develop between the point of maximum velocity and the concave wall.^21-27 The sharp velocity gradient means increase in pressure and the local velocity near the walls is not sufficient to provide complete balance of this pressure gradient.²¹ This imbalance is known as Dean instability and leads to recirculation of fluid in the form of vortices directed from center of the channel towards the outer channel wall and back towards the center, in order to balance the pressure gradient (Fig. 1a).

The pressure and velocity gradient imbalance caused by Dean instability results in vortices/secondary flow defined by a non-dimensional number, called the Dean number (De).^21,23-27 De can be defined as a control parameter for the secondary flow which directly represents the Dean force or force due to secondary flow in curved channels. ^21,22

where Re is the Reynolds number, given by UD_h/ν, U is the average channel velocity, ν is kinematic viscosity, D_h is the hydraulic diameter and R is the radius of the curvature of the convex surface of the curved channel. Hence, the strength of secondary flows is strongly dependent on the dimensions of the channel cross-section and radius of curvature. Note that some other investigations determine the Dean number based on a channel width-length scale, instead of hydraulic diameter.^34,35,38,39

Spiral channel geometry offers a convenient platform to investigate the effects of De and aspect ratio (AR) on secondary flow in curved microchannels. In this work, we used three geometries of Archimedean spiral sorting device with three different aspect ratios (AR), g1 with AR = 0.6(250µm×150µm), g2 with AR = 0.4 (250µm×100µm)  and g3 with AR = 0.2 (500µm×100µm ) (Fig. 2). Here, concave and convex channel surfaces are associated with the smaller dimension of each channel cross section. Convex refers to the inner channel wall and concave refers to the outer channel wall. The onset of instability can be defined by the flow conditions at which De is sufficient to cause the formation of two counter rotating vortices (primary Dean vortices). To determine flow conditions which lead to the onset of Dean instability, we used computational analysis. We simulated the presence of primary Dean vortices and determined the evolution of multiple vortices on increase in De.where Re is the Reynolds number, given by UD_h/ν, U is the average channel velocity, ν is kinematic viscosity, D_h is the hydraulic diameter and R is the radius of the curvature of the convex surface of the curved channel. Hence, the strength of secondary flows is strongly dependent on the dimensions of the channel cross-section and radius of curvature. Note that some other investigations determine the Dean number based on a channel width-length scale, instead of hydraulic diameter.^34,35,38,39

Numerical simulations (Star-CCM+) in Fig. 3a show the velocity gradient at the inlet (section 1) and outlet (section 2) of a single segment of spiral microchannel (250µm×150µm) with the inner radius of 2mm (U = 1m/s). The shift in velocity gradient is also evident from the cross-sectional profiles of the velocity gradient with increase in De, along with the corresponding simulated Dean vectors. At De = 0 (straight section), the center of maximum velocity is at the center of the channel cross-section, leading to no secondary flow and hence, no cross-sectional vectors. At De = 17.2, the center of maximum velocity (red region) shifts towards concave wall leading to imbalance in pressure, thereby causing formation of counter rotating vortices called primary Dean vortices. As De is increased, the region of maximum velocity not only shifts closer to the concave wall, but also increases in area. At De = 172, the maximum of velocity gradient expands near the concave wall and bifurcates, leading to further elongation and extension of the cross-sectional vortex vectors. As the velocity gradient increases due to increase in De, Dean vectors become sharper and begin to shift towards the concave wall which leads to splitting of the primary Dean vortices.  Further increase in De, causes increase in centrifugal force leading to the development of additional regions of pressure gradient near the concave wall. To balance this increase in the pressure gradient, there is formation of additional counter-rotating vortices which locally recirculate the fluid near the concave wall. These vortices are called secondary Dean vortices or additional secondary flow vortices as seen in the Fig. 3 panel for De = 257 and for De = 343.

The secondary flow behavior can be further described using the shift (x) of the center of the maximum velocity from the center of the channel. Fig. 3b shows the plot of this shift relative to half width of the channel (w_0.5). There is a linear increase in the shift till it reaches the secondary vortex regime where the primary vortices start to expand and sharpen and ultimately bifurcate into four vortices. The center of maximum velocity shifts gradually, moving closer to the concave wall, thereby further increasing the pressure gradient and causing the formation of multiple vortices.  These results are comparable with the secondary flow behavior at macro-scale where multiple Dean vortices are observed for high De. In fact, it has been reported that in macrochannels³⁸ these additional vortices develop beyond a critical Dean number (De_C) depending on the aspect ratio of the channel.  Although the simulations computationally confirm the presence of multiple vortices in microchannels, we observed that the shift in focusing position of RBCs occurred at much lower De ~ 37 than indicated by the simulations. Hence, we investigated the development of secondary flow experimentally by means of confocal imaging.

Secondary Dean vortices

In curved macrochannels, a wide variety of methods have been used to study the fluid behavior including dye and smoke contrast and perturbation methods.^20,22,34,36 For example, Dean^21,22 used perturbation methods to study the behavior of Newtonian fluids in curved pipes and Ligrani et al. ^34,35 and Sugiyama et al.^38,39 used smoke contrast in macroscale curved rectangular channels to study Dean flows. These methods are incredibly challenging to perform at microscale. Hence, numerical studies along with simulation have been the most common methods of study of secondary flows in curved microchannels.^32,33 In this work, we used confocal imaging using streamline flow contrast provided by fluorescent dye to image the progression of flow in spiral microchannels at high Re.

For spiral geometry g1 (AR = 0.6), the rate of onset of instability and formation of secondary Dean vortices was investigated. We used confocal microscopy such that the images were taken along both the outer loop, and the inner loop of the spiral microchannel (Fig. 4a). A 1/3^rd confinement of fluorescein dye (1µM) was used to provide contrast for visualizing the streamlines. A constant Re = 200 was maintained as the data was acquired. Near the beginning of curvature, for De = 28.2 (Fig. 4b), because of the onset of the instability, the dye was locally advected by secondary flows which were directed from the convex wall towards the concave wall and pinched at the central section of the channel. Such fluid motion occurred simultaneously as fluid was locally advected from the concave wall towards the convex wall near the edges of the channel. The resulting local variations of static pressure caused recirculation of the dye from the center towards the concave wall, and then, back towards the center of the channel leading to the formation of two primary vortices (Fig. 4 c-d).

As the flow approached the second loop, De increased to 29.4, and recirculation of the flow within the primary Dean vortices was evident near the concave wall (Figs. 4 f-g). Hence, near this location, secondary Dean vortices started to form. Fig. 4g shows formation of the hook shaped vortices, which illustrate the re-distribution of the dye-containing fluid from the concave wall towards the convex wall, followed by recirculation within the primary Dean vortices.  Secondary Dean vortices near the concave wall were observed at De = 30.2 (Fig. 4h) and more clearly at De = 30.6 (Fig. 4i). These vortices developed rapidly as the flow traveled from the first towards the second loop. By the end of the second loop, the dye did not provide sufficient contrast for visualization of vortices, due to molecular diffusion of the dye. Note that local Dean numbers increase as the flow advects through the spiral micro-channel, because of progressively smaller values of R, which result in stronger effects of concave curvature, and locally stronger centrifugal instabilities.

To determine the size of secondary Dean vortices and their trend of development, the portion of channel cross-sectional area occupied by the vortices is presented as it varies with De (Fig. 4 j-l). The plots describe the trend only in one half of the channel, since the vortices form in pairs and the other half is a mirror image. Thus, the maximum vortex area corresponds to 50 percent. The blue data points show the area of the channel covered by one of the two primary Dean vortices, and the red data points show the area of the channel covered by one of the two secondary Dean vortices.  The area of the vortices was measured using image analysis software, ImageJ. The absolute values were then divided by the area of the entire half of the channel to get the percent-coverage of the vortices.  For AR = 0.6, (Fig. 4j) the rapid change of area associated with the primary Dean vortices stabilized at De ~ 29 at approx. 45%. The remaining 5% of the channel cross-section was covered by residual flow. At De > 29.6, secondary Dean vortices started to develop and the area occupied by primary Dean vortices reduced to approximately 25% of the channel. At De ~ 31.25, both the primary and secondary Dean vortices occupied nearly the same area of approximately 15% each. Similar trends of development were observed for channels with lower aspect ratios.

For geometry g2 (AR = 0.4, 250 µm × 100 µm cross-section), the change of area associated with Dean vortices was comparatively lower as is indicated by the gradient of increase in the area of the vortices (Fig. 4k). Re was increased from 200 to 260 to visualize the multiple vortices in the inner loop.  The flow patterns changed in the same manner as for g1, and a pair of secondary Dean vortices was observed for De > De_C. Here, the vortices were somewhat narrow when compared to the primary and secondary Dean vortices associated with g1-geometry. As such, the area covered by the vortices and recirculation of vortices is governed by AR and De as the flow moves downstream.  The data for g2 channel shows that the variation in area occupied by primary Dean vortices has a lower slope (compared to g1 data). Additionally, the size of primary vortices then plateaus for 35< De <36.5. For De>36, we observed a reduction in the area covered by primary Dean vortices with simultaneous development of secondary Dean vortices. For most experimental conditions, the area covered by secondary Dean vortices was observed to be much smaller than the area associated with primary Dean vortices.

For channel geometry g3, the aspect ratio (AR = 0.2) was quite low and the movement of the dye along the channel was very slow leading to a faster molecular diffusion and lower contrast for visualization. However, pinching of the dye at the center of the channel cross-section confirmed the presence of  two primary Dean vortices. At De ~ 38, primary vortices were unable to persist in the same form as they advected downstream, because of local increase in static pressure near the concave wall. This resulted in secondary instability, which in turn, caused the formation of secondary Dean vortices. The secondary Dean vortices were smaller in area when compared to the previous channel geometries, providing additional verification that the strength and shape of the vortices is highly dependent upon AR (Fig. 4l). As such, development of the instabilities associated with Dean flows are also highly dependent upon AR. Additionally, in each case, we observed that beyond a critical De the two primary vortices started recirculating with additional secondary flow to form multiple vortex pairs.

Critical Dean Number

We observed that the flow in spiral microchannels transitions from primary Dean vortices to secondary Dean vortices over a certain threshold of the Dean number, which we termed the critical De (De_c). For De > De_c, the perturbation of primary Dean vortices begins, followed by the development of secondary Dean vortices. At this point, the pressure gradient between the high velocity area and the concave wall has increased to the point that primary Dean vortices are unable to maintain the balance of pressure across the width of the channel. To balance this additional pressure near the concave wall, primary vortices split and recirculate the fluid near the concave wall leading to formation of secondary Dean vortices. De_c denotes the condition associated with decrease in the area covered by primary vortices, and increase in the area covered by secondary vortices. De_c was determined from the results of the experiments in flow visualization for each of the ARs of 0.2, 0.4 and 0.6.

Note that results in Fig. 5 show that different ranges of De are present for the primary and secondary instabilities for each of the channel geometries. For De > De_c, we observed a steady increase in the area covered by secondary vortices until the flow stabilized. We also observed that De_c is highly dependent on the AR (Fig. 5). It was observed that higher the AR, lower is the De_c (De_c α AR^-0.5). This order of dependence is consistent with the classical studies done by Sugiyama et al.^38,39 For AR < 1, Sugiyama’s absolute values of critical De were different, and are in the range of l00-300 (in part, because of a different Dean number definition), but the De_C in his work was also indirectly proportional to AR. His work concluded that the De_c reaches a minimum value when AR nears 1, which agrees with the results presented in this work. The presence of multiple vortices beyond De_c critically affects the focusing of particles and cells in spiral microchannel as discussed in the following section.

Effects on particle focusing

The presence of multiple vortices for De > De_c has important effects upon the focusing of particles and cells in spiral microchannels. Of particular importance are the secondary flows which are associated with secondary Dean vortices, as they affect particle focusing at high Re. Their overall effect is to shift radial positions of the particles from the convex wall towards the concave wall of the channel. This phenomenon was observed using diluted blood samples. Within such curved channels at lower flow rates, RBCs (red blood cells) initially focus closer to the convex wall, as previously reported by the present investigators¹². The cells then shifted towards the concave wall as flow rate increased to 3 mL/min. (channel cross-section = 250µm×100µm). This observation, coupled with confirmation of the presence of secondary Dean vortices near the concave wall, suggested that the cells became entrained within secondary Dean vortices at the higher flow rate values.

To investigate the dynamics of the entrapment, a solution of 10 µm diameter fluorescent polystyrene particles was introduced into a spiral device with AR = 0.4 (g2 geometry) at low De = 17 and high De = 37. It was observed that the particles get trapped in the additional vortices and hence focus near the concave wall (Fig. 6).  This trapping of particles is evident from the fluctuation of the width of the focused stream. At lower De, the particles focus near the inner channel wall and the full width at half maxima (FWHM) of the intensity scan across the channel was ~21 µm. As the flow rate was increased, De increased to 37 and the particles started focusing closer to the concave wall. This focusing position was unsteady and spread over a spatial area. The focusing regime shifted between a narrow focused stream to a broad band. The FWHM of the broad band was 42 µm indicating trapping of the particles in the secondary vortices. To confirm the process of trapping, confocal images were taken at high De and the trapping events were quantified by measuring the intensity of the trapped particles in one of the additional vortices. Fig.6 c shows the stacked image along downstream (x-axis) so that multiple events of trapping can be overlapped to determine the approximate position of the trapped particles. Since the flow rate was very high (~3mL/min), only intensity spikes were obtained rather than complete fluorescent signal. The intensity was measured across the channel at five positions in the bottom half of the channel, spanning one of the lower secondary Dean vortices (Fig. 6d). The intensity peaks at each position describe the subsequent motion of the particles which get trapped within the vortex being measured (Fig. 6e). The intensity peaks show that the particles get trapped in the vortex and recirculate. In fact, the distance of the position of intensity peaks from the concave wall is comparable to the one obtained from the streak velocimetry images (~150-230 µm). From this observation, we can conclude that the particles get trapped in the secondary Dean vortices.

An alternate explanation of this behavior is provided by Toner et al^.40 who denoted the shift of equilibrium positions towards the concave wall to interactions between increased wall-lift forces and Dean forces.  This explanation is generally applicable when particles are located closer to the center of the channel. However, it does not fully explain shift in particle focusing which occur in close proximity to the concave wall.  Overall, this work demonstrates the presence of multiple Dean vortices in low-aspect-ratio, rectangular spiral microchannels, which is consistent with observations at specific Dean numbers in many curved macroscale channels.^34,38,39 These secondary vortices also offer justification for the entrapment of particles, and uncharacteristic focusing of particles, which are observed at higher De and Re.

Discussion

Curvilinear geometry, especially spirals, offer a complex fluidic system which has been used for numerous microfluidic applications. From investigations at macro-scale^21-24 and from numerical investigations at micro-scale^15-20, presence of two counter rotating vortices at lower De has been well established. The presented work, for the first time, demonstrates the presence of multiple pairs of Dean vortices in low-aspect-ratio, rectangular spiral microchannels. The observed sequential development of secondary Dean vortices depends upon the Dean number in the spiral microchannel, as well as the radius of curvature, and the aspect ratio of the channel. Additionally, this vortex development is consistent with the macroscale investigations reported by Sugiyama et al.^38,97 In both macroscale and microscale studies, a dimensionless parameter, critical De (De_c) offers a threshold for the formation of secondary Dean vortices. These secondary Dean vortices develop to balance the additional fluidic pressure near the concave wall of the channel. This additional pressure is generated particularly by increase in Re (increase in volumetric flow rate) as well as on increase in De. These secondary vortices also provide justification for the entrapment of particles, and uncharacteristic focusing of particles, which are observed at higher De.

Even though flow-streamline determination is challenging using cross-sectional visualization images at high Re, confocal images from this work demonstrate presence of the additional secondary Dean vortices within spiral microchannels.  This development directly affects particle focusing within spiral/curved microfluidic devices. As such, the assumption of only two Dean vortex pairs is no longer valid for all experimental conditions, thereby redefining the fluidic model for spiral device design. The present study of the effects of secondary flows and Dean instabilities thus provides new flow modality for manipulation of multiple vortices and other inherent fluid forces to achieve higher efficiency in fluid mixing, particle separation, and heat transfer. This work also paves way into understanding the reason behind the need for a certain range of De for cell focusing in sorting techniques, thereby providing a working window for successful cell sorting in spiral microchannels.

Methods

Geometries.  To investigate flow characteristics and development of the secondary flow, three configurations of an Archimedean spiral with three different aspect ratios (AR) were used.  The details of each geometry were as follows: g1 with AR = 0.6 (250 µm × 150 µm), g2 with AR = 0.4 (250 µm × 100 µm) and g3 with AR = 0.2 (500 µm × 100 µm ). A three inlet system was used to provide 1/3^rd confinement of the dye at the input so that higher contrast was achieved with more time before the dye mixes downstream.  A single outlet was located at the center of each spiral.  Each device is designed with the inner-most radius as 2 mm.

Microfabrication.  Devices were fabricated in polydimethysiloxane (PDMS, Sylgard 184, Dow Corning) using the standard soft lithography process. The 100 µm and 150 µm high masters were formed in SU-8 photoresist (Microchem Corp.).  A mixture of PDMS base and curing agent (10:1 ratio) was poured on the master; after degassing PDMS was cured for 4 h on a 60 °C hotplate.  The cured PDMS devices were peeled off, and inlet/outlet ports were punched with a 14 gauge syringe needle.  PDMS was bonded to standard glass slide using a hand-hold plasma surface treater (BD-20AC, Electro-Technic Products, Inc.).

Device operation and imaging.  We used 1µM fluorescein solution to provide contrast for visualizing the stream lines. A syringe connected to a device with 1/16” peek tubing (Upchurch Scientific) and proper fittings (Upchurch Scientific) provided input flow using a syringe pump (Legota 180, KD scientific).  The flow behavior was first visualized using an inverted epi-fluorescence microscope (IX71, Olympus Inc.) equipped with a 12-bit high-speed CCD camera (Retiga EXi, QImaging).  To image the cross-section, confocal microscopy using Zeiss LSM710 LIVE Duo Confocal Microscope was performed with the similar experimental set-up.  Cross-sectional images were taken at each loop of the spiral starting from the outer-most loop (closest to the inlets) at the interval of 60º and analyzed using Zen lite software along with Image J.

References

1. Gravesen P, Branebjerg J, Jensen OS. Microfluidics – A review. J Micromech Microengineering. 3(4), 168-182, (1993).
2. Beebe DJ, Mensing GA, Walker GM, Physics and applications of microfluidics in biology, Annu Rev Biomed Eng.4, 261-286 (2002).
3. JuliusH., Masuda T., and Herzenberg L. A., Demonstration That Antigen-Binding Cells Are Precursors of Antibody-Producing Cells After Purification with a Fluorescence-Activated Cell Sorter, PNAS. 69(7), 1934–1938, (1972).
4. Schmitz B, Radbruch A, Kümmel T, Wickenhauser C, Korb H, Hansmann ML, Thiele J, and Fischer R., Magnetic activated cell sorting (MACS)-a new immunomagnetic method for megakaryocytic cell isolation: comparison of different separation techniques, Eur J Haematol. 52(5), 267-275 (1994).
5. Zhou, J. & Papautsky, I., Fundamentals of inertial focusing in microchannels. Lab on a Chip. 13, 1121–1130 (2013).
6. Asmolov, E., The inertial lift on a spherical particle in a plane Poiseuille flow at large channel Reynolds number. of Fluid Mech. 381, 63–68 (1999).
7. Bhagat A. A. S., Kuntaegowdanahalli S. S., and Papautsky I., Geometrically modulated inertial microfluidics for continuous particle filtration and extraction, Nanofluid.7, 217-226 (2009).
8. Zhou, J., Giridhar, P. V., Kasper, S. & Papautsky, I. Modulation of aspect ratio for complete separation in an inertial microfluidic channel. Lab on a Chip. 13, 1919–1929 (2013).
9. Di Carlo, D., Inertial microfluidics. Lab on a Chip. 9, 3038–3046 (2009).
10. Kuntaegowdanahalli S. S., Bhagat ,A. A. S., and Papautsky, I., Inertial microfluidics for continuous particle separation in spiral microchannels, Lab Chip. 9, 2973–2980 (2009).
11. Bhagat A. A. S., Kuntaegowdanahalli, S. S. and Papautsky, I., Continuous particles separation in spiral microchannels using Dean flows and inertial migration, Lab Chip. 8, 1906-1914 (2008).
12. Nivedita N, Papautsky I, Continuous separation of blood cells in spiral microfluidic devices, Biomicrofluidics. 7, 054101(1-14) (2013).
13. Gossett D. R., and Di Carlo, D. Particle Focusing Mechanisms in Curving Confined Flows. Analytical Chemistry. 81, 8459–8465 (2009).
14. Guan G, Wu L, Bhagat A.A, Li Z, Chen P C.Y, Chao S, Ong C.J, Han J. Spiral microchannel with rectangular and trapezoidal cross-sections for size based particle separation. Scientific Reports. 3, 1475 (1-9) (2013).
15. Howell P.B., Mott, D.R., Golden J.P., Ligler F.S. Design and evaluation of a Dean vortex-based micromixer. Lab on a Chip. 4(6), 663-9 (2005).
16. Ookawara S, Street D, Ogawa K. Numerical study on development of particle concentration profiles in a curved microchannel. Chemical Engineering Science.61(11), 3714-3724 (2006).
17. Fellouah H, Castelain C, El Moctar AO, Peerhossaini H. A numerical study of Dean instability in non-newtonian fluids. J Fluids Eng Trans ASME. 128(1), 34-41 (2006).
18. Li P, Cogswell J, Faghri M. Design and test of a passive planar labyrinth micromixer for rapid fluid mixing. Sensors and Actuators, B: Chemical. 174, 126-132 (2012).
19. Pathak JS, Ross D, Migler KB. Elastic flow instability, curved streamlines, and mixing in microfluidic flows. Phys Fluids. 16(11), 4028-4034 (2004).
20. Scherr T, Quitadamo C, Tesvich P, Park D. S-W, Tiersch T, Hayes D, Choi J-W, Nandakumar K, Monroe W.T. A planar microfluidic mixer based on logarithmic spirals. J Micromech Microengineering. 22(5), 055019 (1-20) (2012).
21. Dean WR. LXXII. The stream-line motion of fluid in a curved pipe (second paper). Philosophical Magazine Series 7. 5(30), 673-695 (1928).
22. Dean WR. XVI. Note on the motion of fluid in a curved pipe. Philosophical Magazine Series 7. 4(20), 208-223 (1927).
23. Barua SN. On secondary flow in stationary curved pipes. Q J Mech Appl Math. 6(1), 61-77 (1963).
24. Berger SA, Talbot L, Yao LS.Flow in curved pipes. Annu Rev Fluid Mech. 15, 461-512 (1983).
25. Bhunia A, Chen CL. Flow characteristics in a curved rectangular channel with variable cross-sectional area. J Fluids Eng Trans ASME. 131(9), 091102(1-16) (2009).
26. Chandratilleke TT, Ellepola JH, Ho JC. Numerical solution for laminar flow through a curved rectangular duct. American Society of Mechanical Engineers, Fluids Engineering Division (Publication) FED.13, 8p. (1997).
27. Chen JJ, Chen CH, Shie SR. Optimal designs of staggered Dean vortex micromixers. International Journal of Molecular Sciences. 12(6), 3500-3524 (2011).
28. Cheng KC, Akiyama M. Laminar forced convection heat transfer in curved rectangular channels. Int J Heat Mass Transfer. 13(3), 471-490 (1970).
29. Cheng KC, Lin R, Ou J. Fully Developed Laminar Flow in curved rectangular channels.Fluids Eng. 98(1), 41-48 (1976).
30. Dombrowski N, Foumeny EA, Ookawara S, Riza A. Influence of reynolds number on the entry length and pressure drop for laminar pipe flow. Can J Chem Eng. 71(3), 472-476, (1993).
31. Jarrahi M, Castelain C, Peerhossaini H. Secondary flow velocity field in laminar pulsating flow through curved pipes – PIV measurements. Proceedings of the ASME Fluids Engineering Division Summer Conference 2009, FEDSM2009.1(PART C), 1577-1584 (2009).
32. Jiang F, Drese KS, Hardt S, Küpper M, Schönfeld F. Helical flows and chaotic mixing in curved micro channels. AIChE J. 50(9), 2297-2305 (2004).
33. Jitchote W, Robertson AM. Flow of second order fluids in curved pipes. J Non Newtonian Fluid Mech. 90(1), 91-116 (2000).
34. Ligrani PM, Longest JE, Kendall MR, Fields WA. Splitting, merging and span wise wavenumber selection of Dean vortex pairs. Exp Fluids. 18 (1-2), 41-58 (1995).
35. Ligrani PM, Niver RD. Flow visualization of Dean vortices in a curved channel with 40 to 1 aspect ratio. Phys Fluids. 31, 3605-3617 (1988).
36. Schönfeld F, Hardt S. Simulation of helical flows in microchannels. AIChE J. 50(4), 771-778 (2004).
37. Siggers JH, Waters SL. Steady flows in pipes with finite curvature. Phys Fluids. 17(7), 1-18 (2005).
38. Sugiyama S, Hayashi T, Furukawa H. Flows in curved rectangular channels. Nippon Kikai Gakkai Ronbunshu, B Hen/Transactions of the Japan Society of Mechanical Engineers, Part B. 52(484), 3905-3911 (1986).
39. Sugiyama S, Hayashi T, Yamazaki K. Flow characteristics in the curved rectangular channels. Bulletin of the JSME. 26(216), 964-969 (1983).
40. Martel J.M., and Toner M., Particle focusing in curved microfluidic channels, Scientific Reports, 3, 3340, (2013).
41. Sudarshan A.P., Ugaz V.M., Fluid mixing in planar spiral microchannels. Lab Chip, 6(1), 74-82 (2006).

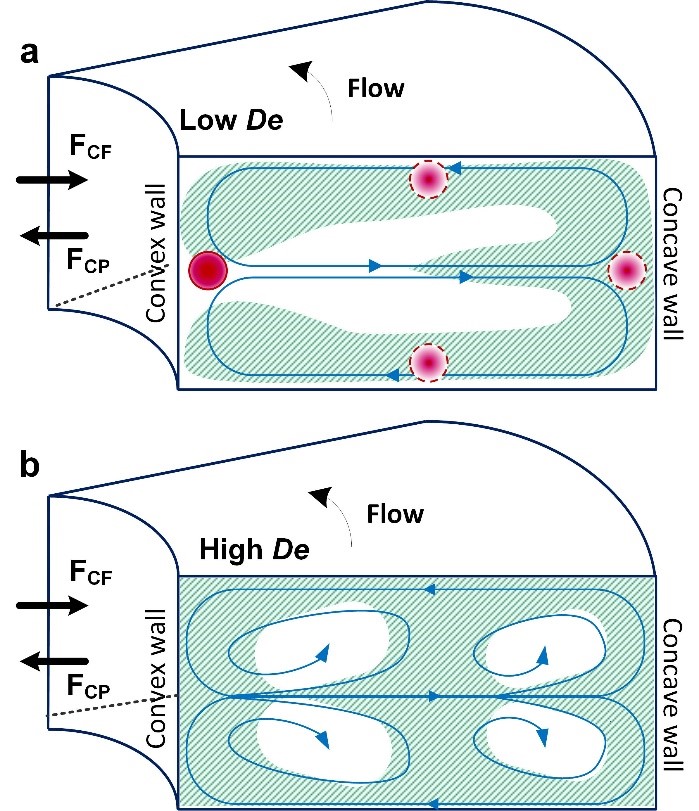
Figure 1. (a) Schematic illustrating two counter rotating vortices in a curved rectangular channel at lower De. It shows the development of primary vortices at lower De caused by the effect of centrifugal (F_CF) and centripetal force (F_CP) on the parabolic velocity profile. (b) Schematic illustrating flow behavior at high De causing the formation of multiple vortices.

Figure 2. Schematic layout of the spiral devices with the cross-sectional dimensions of the three geometries and the respective radii of curvature (convex radius of curvature, R).

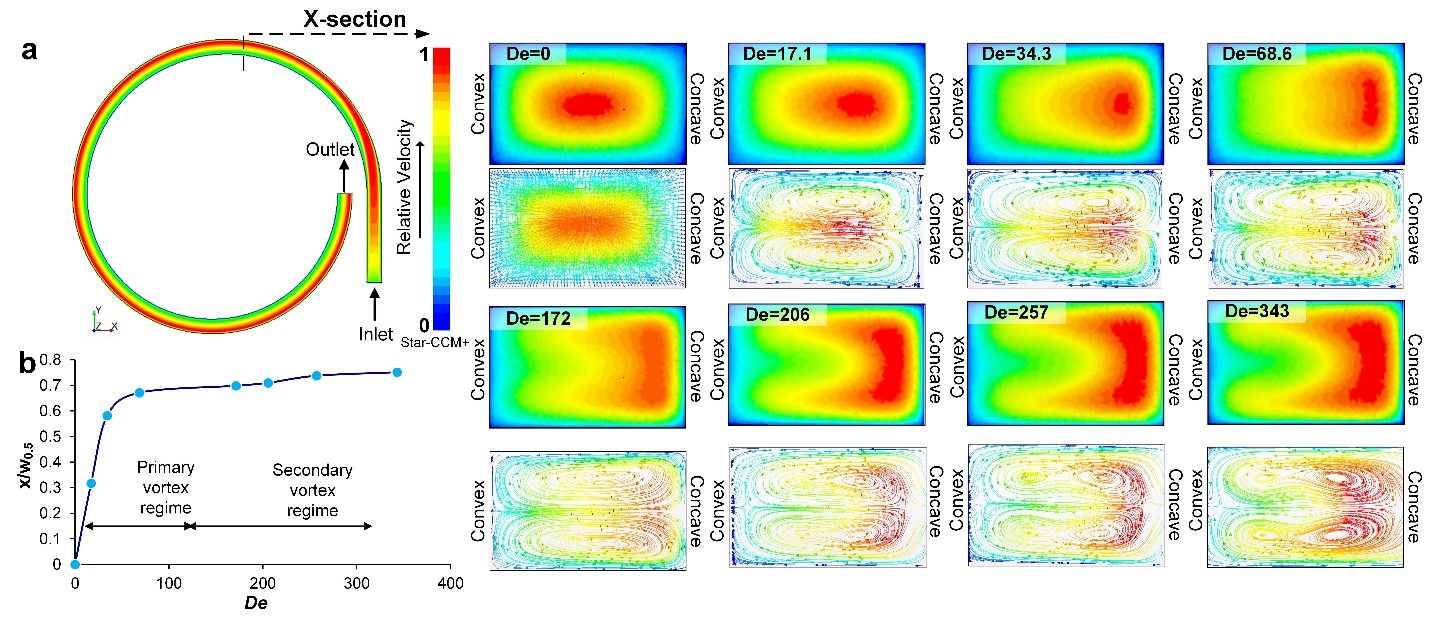
Figure 3. (a) Top-view of the single loop of the spiral model simulated using Star CCM+ at 1 m/s. Cross-sectional images of the scalar velocity profile and corresponding images of the Dean flow vectors from De = 0 (straight channel) and De = 343. (b) Plot of the shift of center of maximum velocity from the center of the channel (x) relative to half width of the channel (W_0.5) as De is increased.

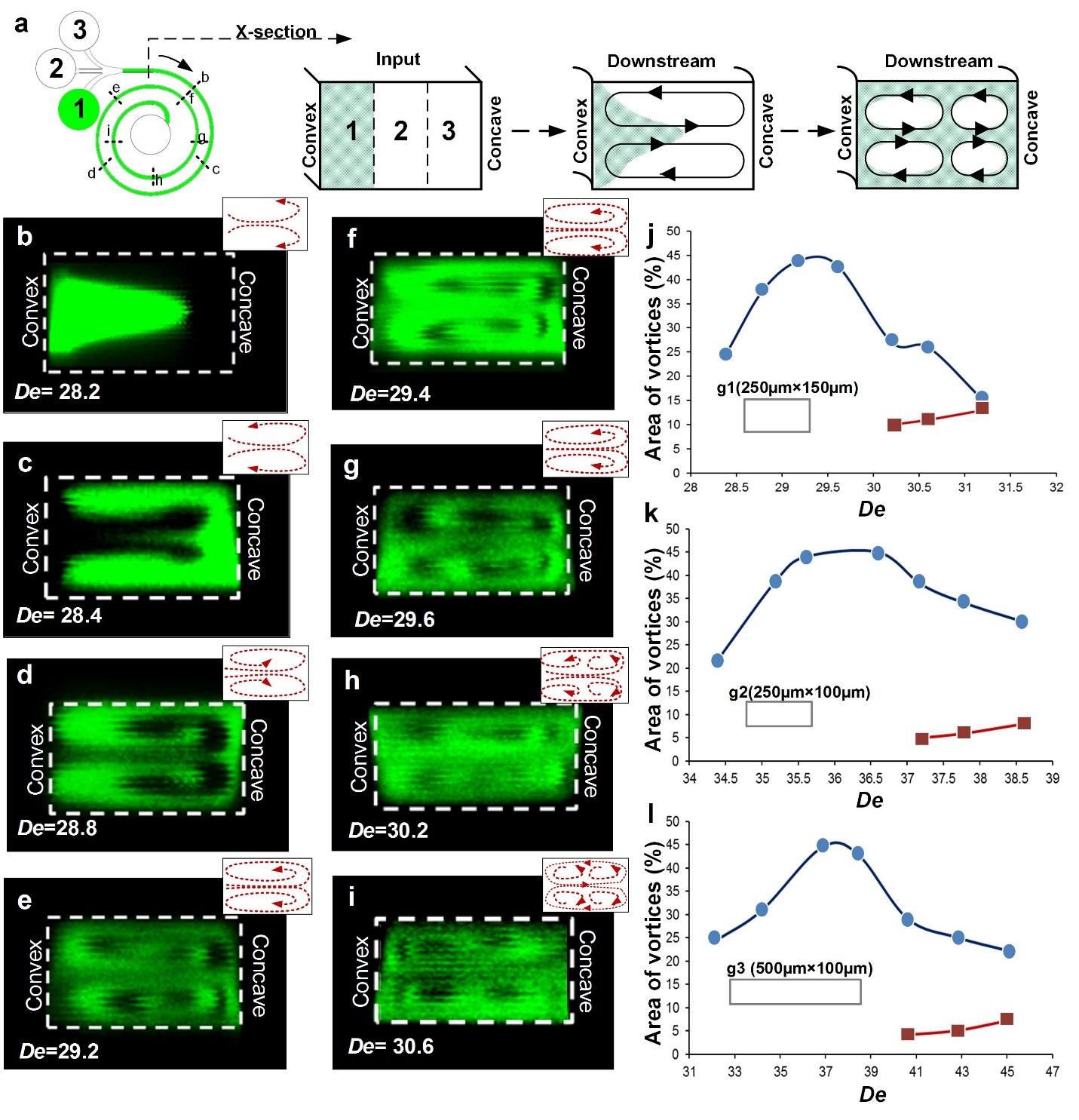
Figure 4 (a) Schematic of 1/3^rd confinement of fluorescein dye to visualize vortex development in spiral micro-channels. (b-i) Confocal images of the cross-section of the rectangular spiral microchannel with geometry g1. The images were taken at regular intervals over two loops. These images show a gradual development of secondary vortices in the second loop of the spiral device whereas in the first loop only primary vortices were observed. (j-l) Plot of Area vs. De for the rectangular spiral microchannel geometries, g1, g2 and g3. The blue data points show the area of the channel covered by one of the two primary vortices, and the red data points show the area of the channel covered by one of the two secondary Dean vortices.

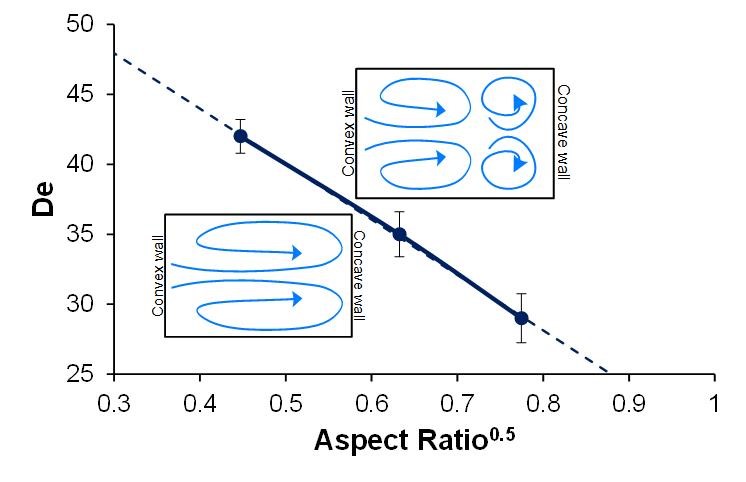
Figure 5 Plot of the critical Dean number as a function of the aspect ratio of the rectangular channel.

Figure 6. (a) Schematic of the process of entrapment of particles in the additional vortices with the insets of the corresponding particle focusing positions and the behavior of RBCs.  (b) Intensity plot of the focused stream of 10 µm diameter particles at low De and trapping at high De. (c) Stacked confocal image of the events involving trapping of particles near the outer channel wall/concave wall. (d) Schematic of the positions where the confocal images were taken to determine the position of trapped 10 µm particles.  (e) Intensity plot across half height and width of the channel to determine the movement of particles in the secondary vortices.